4  Swaps

References
  • HULL, John. Options, futures, and other derivatives. Ninth edition. Harlow: Pearson, 2018. ISBN 978-1-292-21289-0.
    • Chapter 7 - Swaps
  • PIRIE, Wendy L. Derivatives. Hoboken: Wiley, 2017. CFA institute investment series. ISBN 978-1-119-38181-5.
    • Chapter 2 - Basics of Derivative Pricing and Valuation
    • Chapter 3 - Pricing and Valuation of Forward Commitments

Learning Outcomes:

4.1 Nature of Swaps

Definiton

A swap is an over-the-counter (OTC) contract where two parties agree to exchange cash flows at specified intervals based on predetermined terms. Due to its bilateral nature and customization, it carries default risk.

  • Comparison with Forward Contracts: While forward contracts involve a single cash flow exchange at a future date, swaps facilitate multiple exchanges over time, making them more flexible and useful for various financial strategies.

  • Conceptual View: A swap can be seen as a series of bundled forward contracts. This perspective highlights its role in hedging and financing, where cash flow timing and valuation are crucial.

  • Initial Market Value: At inception, a swap has a market value of zero, reflecting a balanced structure where some forward components may hold positive value while others hold negative value. Over time, market fluctuations and underlying asset performance alter the swap’s value.

4.2 Interest Rate Swaps

This section explores a Plain Vanilla Interest Rate Swap, using a transaction between Apple and Citigroup as an example. The swap spans three years and demonstrates the core mechanics of interest rate swaps.

  • Apple agrees to pay Citigroup a fixed 3% annual interest rate, with quarterly payments based on a notional principal of $100 million.

  • In return, Citigroup pays Apple interest at the three-month LIBOR rate, calculated on the same principal.

  • Apple is the fixed-rate payer, while Citigroup is the floating-rate payer.



The table below illustrates potential cash flow outcomes, maintaining the $100 million notional principal. The net cash flow for Apple varies due to LIBOR fluctuations, sometimes resulting in payments and other times in receipts.

Date SOFR Rate (%) Floating Cash Flow Received ($’000s) Fixed Cash Flow Paid ($’000s) Net Cash Flow ($’000s)
June 8, 2022 2.20 550 750 -200
Sept. 8, 2022 2.60 650 750 -100
Dec. 8, 2022 2.80 700 750 -50
Mar. 8, 2023 3.10 775 750 +25
June 8, 2023 3.30 825 750 +75
Sept. 8, 2023 3.40 850 750 +100
Dec. 8, 2023 3.60 900 750 +150
Mar. 8, 2024 3.80 950 750 +200

Typical Applications of Interest Rate Swaps

Interest rate swaps are widely used for managing interest rate risk, allowing firms to adjust their exposure by switching between fixed and floating rates based on financial strategy and market outlook.

  • Apple Converts a Floating-Rate Liability to Fixed:


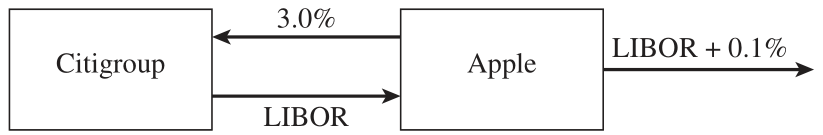
  • Intel Converts a Fixed-Rate Liability to Floating:


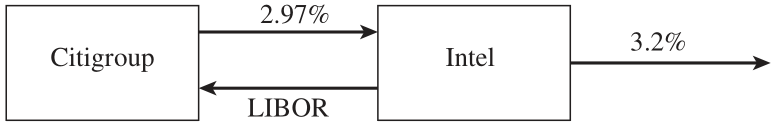
  • Apple Converts a Fixed-Rate Asset to Floating:


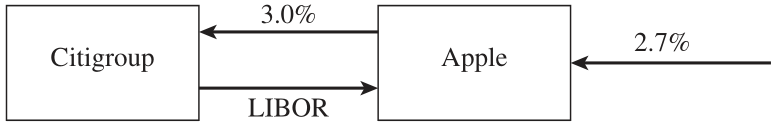
  • Intel Converts a Floating-Rate Asset to Fixed:


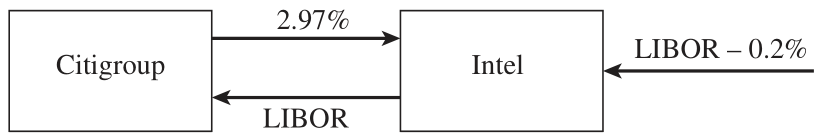
4.3 The Comparative-Advantage Argument

The comparative-advantage argument explains why companies engage in interest rate swaps to optimize borrowing costs. It highlights how firms can leverage differences in borrowing rates to achieve more favorable financing conditions.

Case Study: AAACorp and BBBCorp

Consider two hypothetical companies:

  • AAACorp prefers floating-rate borrowing.
  • BBBCorp prefers fixed-rate borrowing.

Their respective borrowing costs are:

Entity Fixed Rate Floating Rate
AAACorp 4.0% Floating - 0.1%
BBBCorp 5.2% Floating + 0.6%
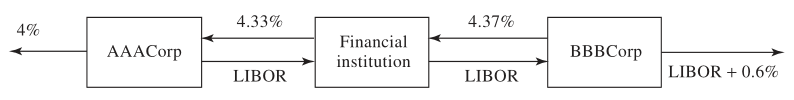
  • Direct Swap Mechanism: AAACorp borrows at its preferred floating rate, while BBBCorp borrows at its preferred fixed rate. They swap obligations, effectively allowing each company to access the preferred rate indirectly.


  • Indirect Swap via a Financial Institution: A financial institution can act as an intermediary, structuring the swap between AAACorp and BBBCorp. This setup reduces negotiation complexities and often enhances efficiency.


Criticism of the Comparative-Advantage Argument

While this argument supports the rationale for interest rate swaps, it has limitations:

Mismatch in Rate Terms:

  • Fixed rates (e.g., 4.0% for AAACorp and 5.2% for BBBCorp) apply to 5-year loans.
  • Floating rates (e.g., Floating - 0.1% for AAACorp and Floating + 0.6% for BBBCorp) are based on 6-month terms.
  • This disparity complicates direct comparisons of borrowing advantages.

Future Rate Uncertainty:

  • BBBCorp’s future borrowing costs depend on the spread over the floating rate at each reset.
  • If interest rates fluctuate significantly, BBBCorp may face unexpected financial exposure.

4.4 Valuation of Interest Rate Swaps

Interest rate swaps are used to manage interest rate exposure, and their value fluctuates as market conditions change. At inception, a swap’s value is approximately zero, reflecting an initial balance between fixed and floating cash flows. Over time, valuation techniques—particularly those using Forward Rate Agreements (FRAs)—help determine its market value.

Valuation Framework

The swap valuation process consists of three key steps:

  1. Calculate Floating Forward Rates: Use market data to estimate future floating interest rates, which determine floating-rate payments.

  2. Determine Swap Cash Flows: Apply calculated forward rates to estimate future cash flows for both fixed and floating legs of the swap.

  3. Discount Cash Flows to Present Value: Discount future cash flows using Overnight Indexed Swap (OIS) rates, adjusting for time value and credit risk.

Swap Agreement Overview

  • Structure: A swap where one party pays a fixed annual rate of 3% and receives SOFR semi-annually on a $100 million principal.
  • Remaining Term: 1.2 years, with cash flow exchanges at 0.2, 0.7, and 1.2 years.
  • Risk-Free Rates (OIS zero rates for different maturities):
    • 3 months: 2.8%
    • 9 months: 3.2%
    • 15 months: 3.4%
  • Historical SOFR Rate: 2.9% (already fixed for the upcoming 3-month exchange).
  • Projected Forward SOFR Rates:
Time (years) Continuous Compounding Semi-Annual Compounding
0.2 2.50% 2.516%
0.7 3.36% 3.388%
1.2 3.68% 3.714%

Cash Flow Valuation

The table below details the swap’s fixed and floating cash flows, their net impact, and their present value after discounting.

Time (years) Fixed Cash Flow ($M) Floating Cash Flow ($M) Net Cash Flow ($M) Discount Factor Present Value ($M)
0.2 -1.500 +1.258 -0.242 0.9944 -0.241
0.7 -1.500 +1.694 +0.194 0.9778 +0.190
1.2 -1.500 +1.857 +0.357 0.9600 +0.343
Total +0.292

  • Fixed Cash Flow (at 0.7 years):
    \[ 0.5 \times 0.03 \times 100 = -1.5 \text{ million} \]

  • Floating Cash Flow (based on 3.388% semi-annual rate for 0.7 years):
    \[ 0.5 \times 0.03388 \times 100 = 1.694 \text{ million} \]

  • Present Value of Net Cash Flow (discounting at OIS rate for 0.7 years):
    \[ 0.194 \times e^{-0.032 \times 0.7} = 0.190 \text{ million} \]

  • Final Swap Value:
    The sum of all present values across time points, resulting in a swap valuation of $0.292 million.

  • This valuation assumes simplifications (e.g., ignoring holiday calendars and day count conventions), which may affect precise cash flows.

4.5 Currency Swaps

Currency swaps are financial instruments that enable the exchange of principal and interest payments in different currencies between two parties. These contracts help firms manage currency exposure, reduce borrowing costs, and optimize investments across global markets.

In a fixed-for-fixed currency swap, parties exchange fixed interest rate payments in one currency for fixed payments in another currency, along with the principal exchange at the start and end of the contract.

Currency swaps are widely used for:

  1. Liability Management: Firms convert liabilities from one currency to another to match their currency exposure and reduce exchange rate risk.

  2. Investment Optimization: Investors swap investment returns between currencies to access favorable interest rates or hedge against currency fluctuations.

Agreement Overview

  • Parties Involved: British Petroleum and Barclays.
  • Term: 5 years.
  • Structure:
    • British Petroleum pays a fixed 3% interest rate in USD.
    • British Petroleum receives a fixed 4% interest rate in GBP.
  • Principal Exchange:
    • USD 15 million exchanged for GBP 10 million at inception.
    • Principal amounts are re-exchanged at the end of the swap.
  • Interest Payments: Made annually, based on the fixed rates in each currency.

Yearly Cash Flow Summary

The table below illustrates the cash flows for British Petroleum exchanged over the swap’s duration:

Date USD Cash Flow (millions) GBP Cash Flow (millions)
Feb 1, 2022 +15.00 -10.00
Feb 1, 2023 -0.45 +0.40
Feb 1, 2024 -0.45 +0.40
Feb 1, 2025 -0.45 +0.40
Feb 1, 2026 -0.45 +0.40
Feb 1, 2027 -15.45 +10.40

Comparative Advantage in Currency Swaps

A comparative advantage in currency swaps arises when companies face different borrowing costs in various currencies due to taxation, credit ratings, or market conditions.

  • Efficiency: Currency swaps enable companies to lower borrowing costs and manage currency risk.
  • Strategic Use: They help convert liabilities and investments across different currencies.
  • Comparative Advantage: Firms leverage differential borrowing costs to gain financial benefits.
  • Scenario:
    • General Electric (GE) wants to borrow in Australian dollars (AUD).
    • Qantas Airways wants to borrow in U.S. dollars (USD).
  • Borrowing Costs (after tax adjustments):
USD AUD
General Electric 5.0% 7.6%
Qantas Airways 7.0% 8.0%

Since General Electric has a lower borrowing cost in USD and Qantas Airways has a lower borrowing cost in AUD, they can swap their borrowing obligations to access cheaper rates than they would get individually.



4.6 Valuation of Fixed-for-Fixed Currency Swaps

Fixed-for-fixed currency swaps involve exchanging fixed interest payments in two different currencies over a set period, alongside an initial and final principal exchange. These swaps can be viewed as a series of forward foreign exchange contracts, where future exchange rates determine the valuation of expected cash flows.

The core assumption in valuing these swaps is that forward exchange rates reflect the market’s expectations of future currency values. The valuation process, therefore, involves discounting cash flows in each currency and converting them using forward exchange rates.

  • Forward Exchange Rates Matter: The swap’s valuation depends on market expectations of future exchange rates.
  • Discounting Reflects Present Value: Future cash flows must be discounted using the domestic interest rate to obtain today’s value.
  • Principal Exchange is Crucial: The final principal repayment significantly impacts the swap’s overall valuation.

Consider a U.S. dollar (USD) - Japanese yen (JPY) swap with the following terms:

  • Fixed Interest Rates:
    • USD: 2.5% per annum (continuous compounding).
    • JPY: 1.5% per annum (continuous compounding).
  • Swap Payments:
    • Receives 3% interest in JPY.
    • Pays 4% interest in USD.
  • Principal Exchange:
    • USD 10 million exchanged for 1,200 million JPY at inception and at maturity.
  • Remaining Swap Duration: 3 years.
  • Current Spot Exchange Rate: 110 JPY per USD.

The table below outlines the expected cash flows in both currencies, their conversion using forward exchange rates, and their discounted present values:

Time (years) Dollar Cash Flow (million) Yen Cash Flow (million) Forward Exchange Rate Dollar Value of Yen Cash Flow (million) Net Cash Flow (million) Present Value (million)
1 -0.4 +36 0.009182 0.3306 -0.0694 -0.0677
2 -0.4 +36 0.009275 0.3339 -0.0661 -0.0629
3 -10.4 +1236 0.009368 11.5786 +1.1786 +1.0934
Total +0.9629

  • USD Payment:
    \[ 0.04 \times 10 = -0.4 \text{ million USD per year} \]

  • JPY Receipt:
    \[ 1,200 \times 0.03 = +36 \text{ million JPY per year} \]

  • Principal Exchange at Maturity (Year 3):

    • Pays: USD 10 million
    • Receives: 1,200 million JPY

Forward Exchange Rate Calculations

The valuation relies on forward exchange rates, derived from the interest rate differential between USD and JPY:

\[ F_t = S_0 \times e^{(r - r_f) \times t} \]

  • \(S_0 = 0.009091\) (initial spot rate, 1/110 JPY per USD).
  • \(r = 2.5\%\) (USD interest rate).
  • \(r_f = 1.5\%\) (JPY interest rate).
  • \(t\) = time in years.

Forward Rates for Each Year

  • Year 1:
    \[ 0.009091 \times e^{(0.025 - 0.015) \times 1} = 0.009182 \]
  • Year 2:
    \[ 0.009091 \times e^{(0.025 - 0.015) \times 2} = 0.009275 \]
  • Year 3:
    \[ 0.009091 \times e^{(0.025 - 0.015) \times 3} = 0.009368 \]

Valuation Process

  1. Convert Future JPY Cash Flows to USD Using Forward Rates
    • Example (Year 1):
      \[ 36 \times 0.009182 = 0.3306 \text{ million USD} \]
    • Net Cash Flow (Year 1):
      \[ 0.3306 - 0.4 = -0.0694 \text{ million USD} \]
  2. Discount Net Cash Flows Using USD Interest Rate (2.5%)
    • Example (Year 1):
      \[ -0.0694 \times e^{-0.025 \times 1} = -0.0677 \text{ million USD} \]
  3. Repeat for Years 2 and 3
    • The same process applies for each year’s cash flows, using the respective forward rates and discount factors.
  4. Sum Present Values to Obtain Swap Valuation
    • The total present value of net cash flows is $0.9629 million USD, representing the swap’s market value.

4.7 Other Currency Swaps

Fixed-for-Floating Currency Swaps

A fixed-for-floating currency swap combines elements of a fixed-for-fixed currency swap and a fixed-for-floating interest rate swap. In this arrangement, one party pays a fixed interest rate in one currency while receiving a floating interest rate in another currency.

Consider a swap agreement where:

  • A party pays a floating interest rate on GBP 7 million.
  • The same party receives a fixed 3% interest rate on USD 10 million, with semiannual payments over 10 years.

This swap can be broken down into two components:

  1. Currency Swap Component:
    • Receives 3% fixed interest on USD 10 million.
    • Pays 4% fixed interest on GBP 7 million.
  2. Interest Rate Swap Component:
    • Receives 4% fixed interest.
    • Pays floating sterling interest on GBP 7 million.

Floating-for-Floating Currency Swaps

A floating-for-floating currency swap involves exchanging floating interest payments in two different currencies. It effectively combines a fixed-for-fixed currency swap with two floating interest rate swaps.

A party exchanges:

  • Sterling floating interest payments on GBP 7 million.
  • For dollar floating interest payments on USD 10 million.

This structure consists of:

  1. Base Swap:
    • Pays 3% fixed interest on USD 10 million.
    • Receives 4% fixed interest on GBP 7 million.
  2. Interest Rate Swap #1:
    • Pays 4% fixed interest.
    • Receives floating sterling interest on GBP 7 million.
  3. Interest Rate Swap #2:
    • Pays 3% fixed interest.
    • Receives floating USD interest on USD 10 million.

4.8 Other Types of Swaps

Beyond currency and interest rate swaps, financial markets offer specialized swaps tailored for risk management, investment strategies, and arbitrage opportunities.

  • Amortizing/Step-Up Swap: The notional principal gradually increases or decreases to match an underlying asset or liability.

  • Compounding Swap: Interest payments are reinvested, compounding over the swap’s duration.

  • Constant Maturity Swap (CMS): The interest rate resets periodically based on the rate of a constant maturity instrument (e.g., a 10-year Treasury note).

  • LIBOR-in-Arrears Swap: The interest rate is determined at the end of the payment period instead of the beginning, increasing uncertainty.

  • Accrual Swap: Interest accrues only when a benchmark rate (or index) meets specific conditions.

  • Equity Swap: Exchanges equity returns (e.g., stock performance) for either fixed or floating interest payments.

  • Cross-Currency Interest Rate Swap: A variation of currency swaps where one or both legs involve floating rates in different currencies.

  • Diff Swap: An interest rate differential swap, where payments depend on the difference between two reference rates.

  • Commodity Swap: One party pays a fixed price for a commodity, while the other pays the floating market price over time.

  • Variance Swap: A contract based on future volatility, where payments depend on the variance of an underlying asset’s price.

4.9 Practice Questions and Problems

  1. A bank finds that its assets are not matched with its liabilities. It is taking floating-rate deposits and making fixed-rate loans. How can swaps be used to offset the risk?

  2. Explain the difference between the credit risk and the market risk in a financial contract.

  3. Explain why a bank is subject to credit risk when it enters into two offsetting swap contracts.

  4. Why is the expected loss from a default on a swap less than the expected loss from the default on a loan to the same counterparty with the same principal?

  5. A corporate treasurer tells you that he has just negotiated a five-year loan at a competitive fixed rate of interest of 5.2%. The treasurer explains that he achieved the 5.2% rate by borrowing at six-month LIBOR plus 150 basis points and swapping LIBOR for 3.7%. He goes on to say that this was possible because his company has a comparative advantage in the floating-rate market. What has the treasurer overlooked?

  6. Companies A and B have been offered the following rates per annum on a $20 million five-year loan:

Fixed Rate Floating Rate
Company A 5.0% Floating + 0.1%
Company B 6.4% Floating + 0.6%

Company A requires a floating-rate loan; company B requires a fixed-rate loan. Design a swap that will net a bank, acting as intermediary, 0.1% per annum and that will appear equally attractive to both companies.

  1. Company X wishes to borrow U.S. dollars at a fixed rate of interest. Company Y wishes to borrow Japanese yen at a fixed rate of interest. The amounts required by the two companies are roughly the same at the current exchange rate. The companies have been quoted the following interest rates, which have been adjusted for the impact of taxes:
Yen Dollars
Company X 5.0% 9.6%
Company Y 6.5% 10.0%

Design a swap that will net a bank, acting as intermediary, 50 basis points per annum. Make the swap equally attractive to the two companies and ensure that all foreign exchange risk is assumed by the bank.

  1. Companies X and Y have been offered the following rates per annum on a $5 million 10-year investment:
Fixed Rate Floating Rate
Company X 8.0% Floating
Company Y 8.8% Floating

Company X requires a fixed-rate investment; company Y requires a floating-rate investment. Design a swap that will net a bank, acting as intermediary, 0.2% per annum and will appear equally attractive to X and Y.

  1. A $100 million interest rate swap has a remaining life of 10 months. Under the terms of the swap, six-month LIBOR is exchanged for 7% per annum (compounded semiannually). The average of the bid-offer rate being exchanged for six-month LIBOR in swaps of all maturities is currently 5% per annum with continuous compounding (5.063% with semi-annual compounding). The six-month LIBOR rate was 4.6% per annum two months ago. What is the current value of the swap to the party paying floating? What is its value to the party paying fixed?

  2. A currency swap has a remaining life of 15 months. It involves exchanging interest at 10% on 20 million GBP for interest at 6% on 30 million USD once a year. The term structure of interest rates in both the United Kingdom and the United States is currently flat, and if the swap were negotiated today the interest rates exchanged would be 4% in dollars and 7% in sterling. All interest rates are quoted with annual compounding (the continuously compounded interest rates in sterling and dollars are 6.766% per annum and 3.922% per annum). The current exchange rate (dollars per pound sterling) is 1.5500. What is the value of the swap to the party paying sterling? What is the value of the swap to the party paying dollars?